naxyard.blogg.se - T standard normal table

Then compare the blue curve with 10 degrees of freedom to the green curve for the z-distribution. The t-distribution with one degree of freedom is shorter and has thicker tails than the z-distribution. Compare the pink curve with one degree of freedom to the green curve for the z-distribution. You can see how the curves with more degrees of freedom are more like a z-distribution. All three t-distributions have “heavier tails” than the z-distribution. The curves with more degrees of freedom are taller and have thinner tails. The shape of the t-distribution depends on the degrees of freedom.

As the sample size increases, the t-distribution becomes more similar to a normal distribution.Ĭonsider the following graph comparing three t-distributions with a standard normal distribution:Īll of the distributions have a smooth shape.

The t-distribution is most useful for small sample sizes, when the population standard deviation is not known, or both.

The t-distribution is defined by the degrees of freedom.

The t-distribution does not make this assumption.

The normal distribution assumes that the population standard deviation is known.

Like a standard normal distribution (or z-distribution), the t-distribution has a mean of zero.

If you think about folding it in half at the mean, each side will be the same.

Like the normal distribution, the t-distribution is symmetric.

Like the normal distribution, the t-distribution has a smooth shape.Instead of diving into complex math, let’s look at the useful properties of the t-distribution and why it is important in analyses. It has a precise mathematical definition. Thank you in advance for any help you can provide.The t-distribution is similar to a normal distribution. Could anyone tell me how to know (from questions) whether to use z or use t? And please show me the differences between 2 exercises here that make one use z-value and the other use t-value. The 1st one use z-table and the 2nd use t-table. I look at the solution my teacher provided.

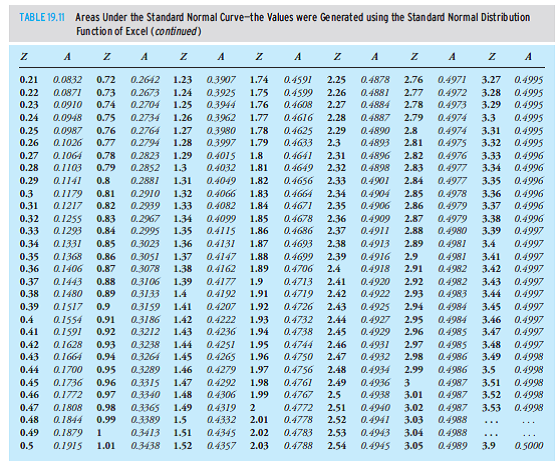
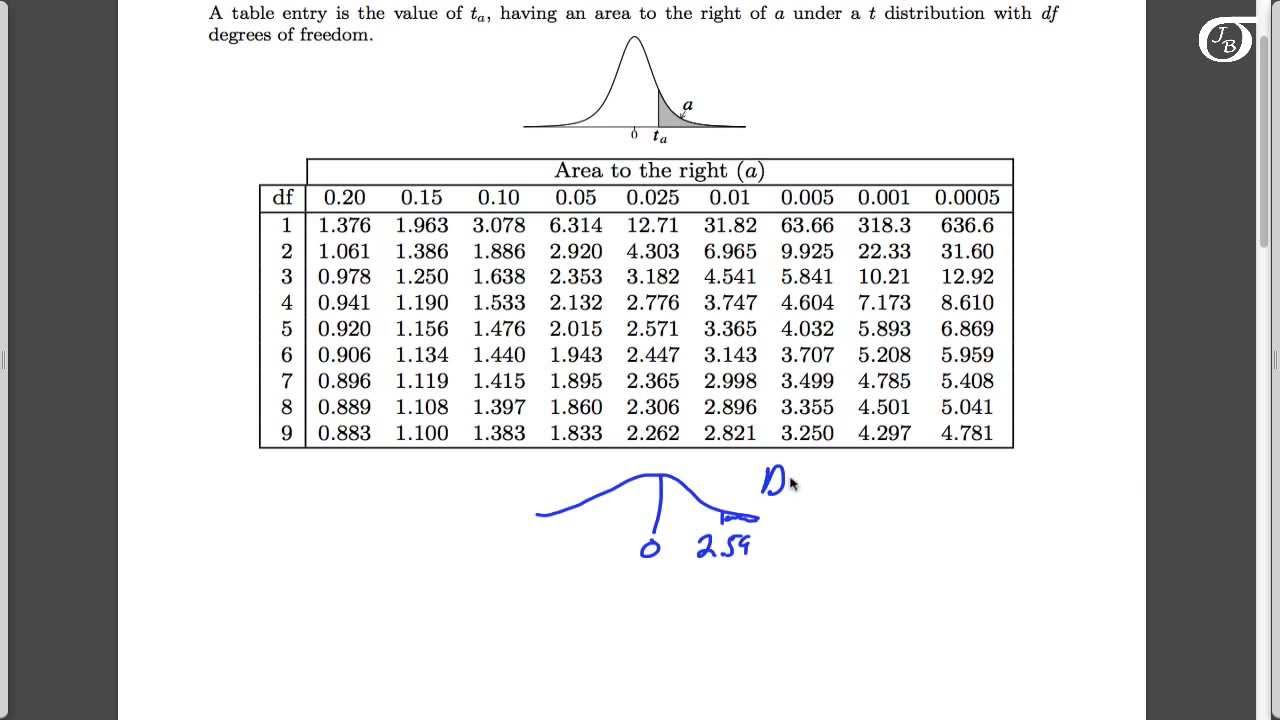
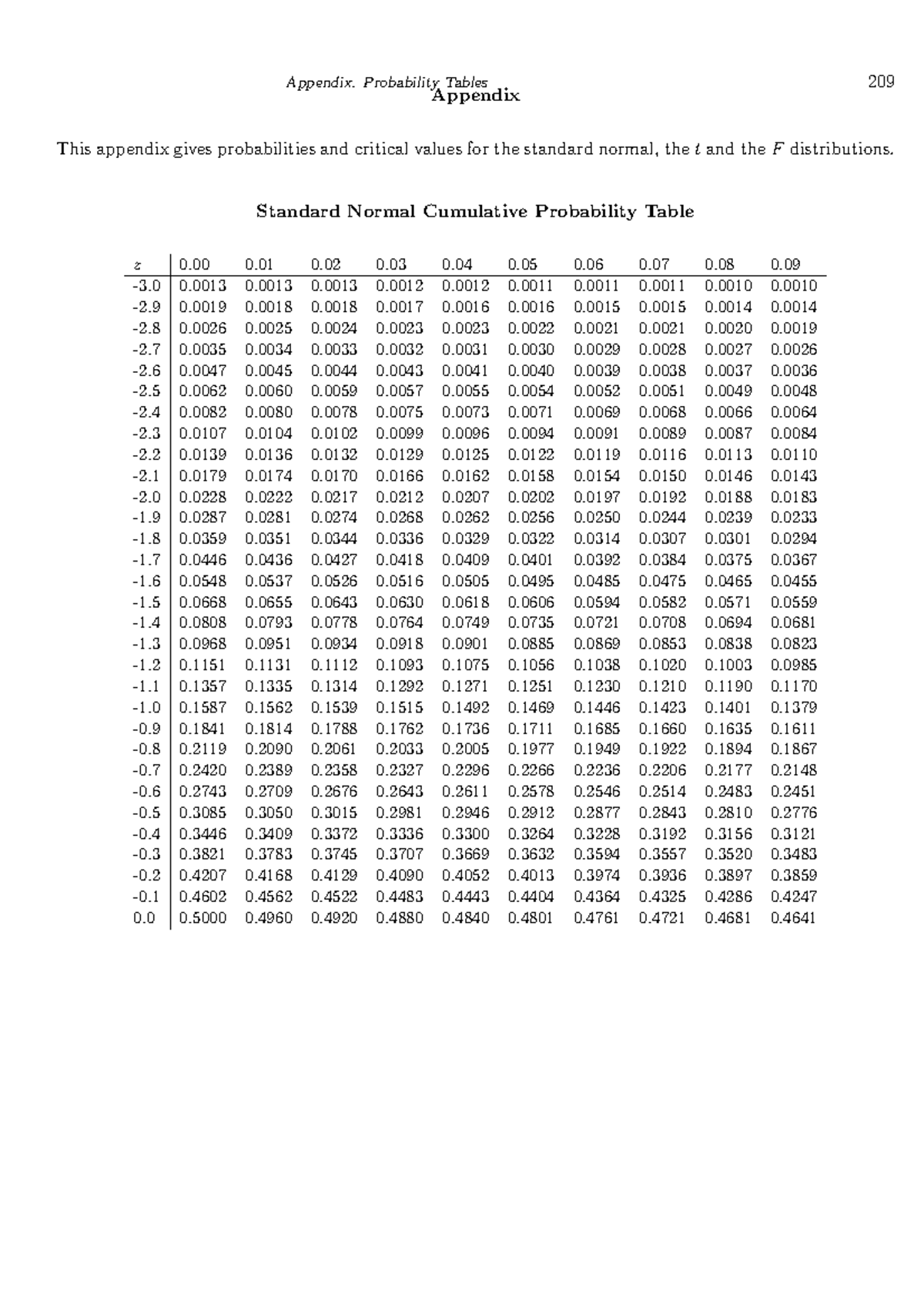
Assume that theĭistribution of the calorie content is approximately normal. Interval for the true mean calorie content of this brand of energy bar. The 2nd: A random sample of 10 chocolate energy bars of a certain brand has, on average, 230Ĭalories per bar, with a standard deviation of 15 calories. If a sample of 30īulbs has an average life of 780 hours, find a 96% confidence interval for the population The 1st: An electrical firm manufactures light bulbs that have a length of life that is approximately normally distributed with a standard deviation of 40 hours.